Right Triangle Trigonometry part 2

Instructor Katherine Cliff

Warm up: Simplify the following expression if possible.

\[\frac{3+6x}{x+2x^2}\]

A. \(3x\)

B. Not possible

C. \(\frac{3}{x}\)

D. \(\frac{3+3x}{x+2x^2}\)

Announcements/reminders



Grades and feedback in myopenmath

Exam 1 in two weeks!

plickers

Example: Calculate sin⁡(α), cos⁡(α), and tan⁡(α) given the following triangle:

plickers

Work on number 1 in your packet.

Consider the equilateral triangle pictured below:

When we draw an altitude connecting the top corner to the base of the triangle, we’ve created our first special right triangle, a \(30^\circ-60^\circ-90^\circ\) triangle. Use the Pythagorean Theorem to solve for the height of the triangle, then use this triangle to find the sine and cosine of \(30^\circ\) and the sine and cosine of \(60^\circ\).

Consider the equilateral triangle pictured below:

Special Right Triangle

Use what you’ve learned to find the sines and cosines of \(\frac{\pi}{6}\) and \(\frac{\pi}{3}\).

Now work on numbers 3 and 4 in the packet.

We’ve created our second special right triangle, a \(45^\circ-45^\circ-90^\circ\) triangle. Use it to find the sine and cosine of \(45^\circ\).

Now use what you’ve learned to find the sine and cosine of \(\frac{\pi}{4}\).

Reciprocal Trigonometric Ratios







Important note about the reciprocal ratios!!!!

Example:

Calculate csc⁡(α), sec⁡(α), and cot⁡(α) given the following triangle:

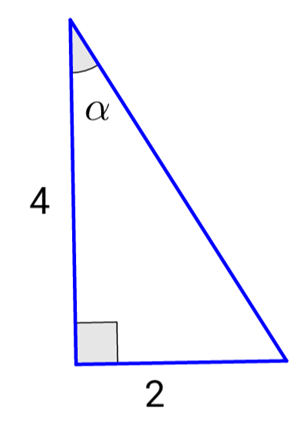
Example:

Calculate csc⁡(α), sec⁡(α), and cot⁡(α) given the following triangle:

plickers

Using Right Triangle Trig to solve for missing information.

What do we do if we don’t have a special triangle?

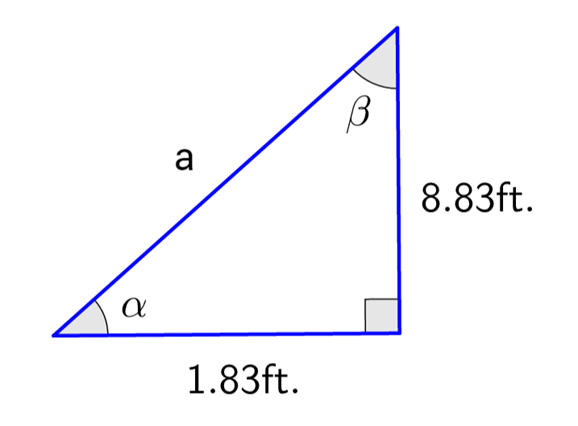
Example: Find the value of a

Example: Find the value of a

plickers

Example: Find the value of x

plickers

Inverse Trigonometric Functions

In a right triangle with reference angle \(\theta\)…

  • If \(\sin(\theta) = n\), then \(\sin^{-1}(n) = \theta\) or \(\arcsin(n)=\theta\).



  • If \(\cos(\theta) = n\), then \(\cos^{-1}(n) = \theta\) or \(\arccos(n)=\theta\).



  • If \(\tan(\theta) = n\), then \(\tan^{-1}(n) = \theta\) or \(\arctan(n)=\theta\).



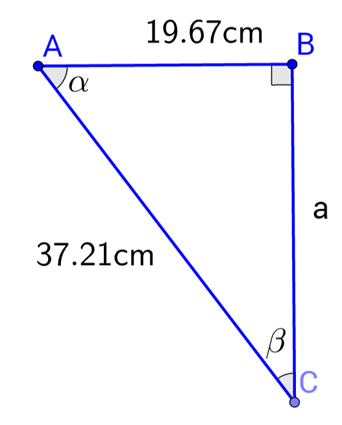
Example: Solve for \(\alpha\)

Example: Solve for \(\beta\)

plickers




A. \(\arccos\left(\frac{19.67}{37.21}\right)\)

B. \(\arcsin\left(\frac{19.67}{37.21}\right)\)

C. \(\frac{1}{\sin\left(\frac{19.67}{37.21}\right)}\)

D. \(\frac{1}{\cos\left(\frac{19.67}{37.21}\right)}\)

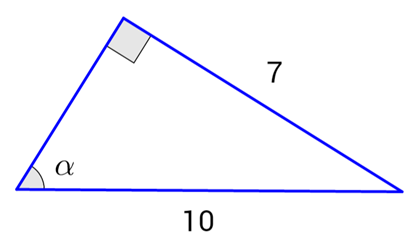
Example: Solve for \(\alpha\)

plickers